Pass Numerical Reasoning
Numerical Reasoning Test
Register for Free Tests
Revise Each Topic
Book a Tuition Session
What We Do
Pass Numerical Reasoning provides comprehensive support for candidates preparing for numerical reasoning tests. Our online course covers essential topics such as percentages, ratios, averages, and data interpretation, helping users develop the skills needed for real numerical reasoning assessments. We also offer free numerical reasoning tests to help candidates familiarise themselves with test formats and improve accuracy under timed conditions. In addition, our free numerical reasoning revision pages provide detailed explanations and step-by-step solutions, ensuring a strong understanding of key mathematical concepts. Whether you’re preparing for a graduate scheme, apprenticeship, or professional role, Pass Numerical Reasoning gives you the tools to succeed.

Why Choose the Pass Numerical Reasoning Service?
Numerical Test Preparation
Revise and prepare with Pass Numerical Reasoning, from revision, to practice questions and full mock tests.
Full Online Course
We provide a comprehensive online course for graduates preparing for numerical reasoning assessments.
Free Numerical Reasoning Tests
Try our free numerical reasoning tests to familiarise yourself with the types of questions you may face.
Numerical Reasoning Tuition
Book a numerical reasoning tutor to help you with certain topics you may struggle with.
Free Numerical Reasoning Tests
Give yourself the best chance of success by becoming familiar with the format and common question types used in numerical reasoning tests. One of the most effective ways to prepare is by trying our free numerical reasoning practice questions and answers. Our free online test includes 20 questions and takes 20 minutes to complete, mirroring the style and timing of the assessments you’re likely to encounter during the application process. The questions are based on real workplace scenarios and test your ability to work with percentages, ratios, and data interpretation. To perform well, take your time to read each question carefully, check your workings, and manage the time available. Once you’ve completed the test, you’ll receive detailed written solutions and video walkthroughs to help you understand the correct answers.
Sign up now for free access to our online numerical reasoning practice tests and get the preparation you need to succeed.
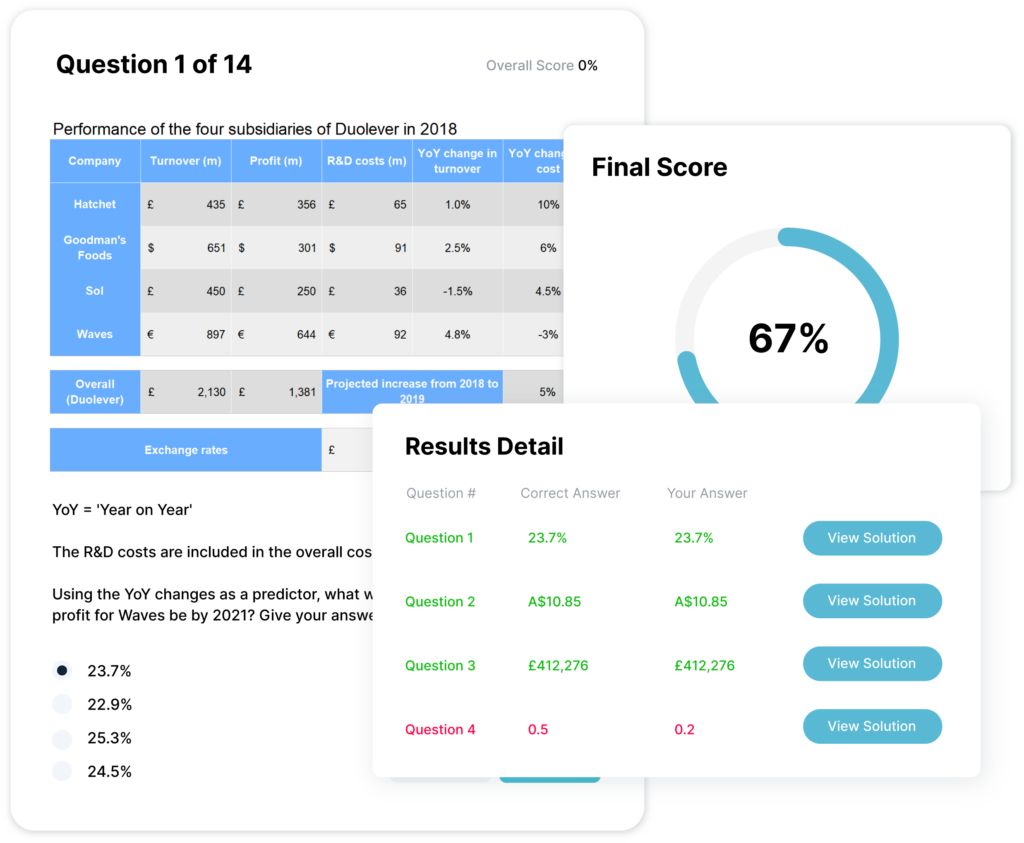
Free Numerical Reasoning Revision
Revising for your numerical reasoning test is a key part of ensuring you perform at your best. With a range of free resources available, including practice tests and revision materials, you can focus on the most important topics that regularly appear in assessments. These include core number skills such as percentages, ratios, fractions, and compound growth, as well as data-handling topics like bar charts, pie charts, and line graphs. You can also practise interpreting information from tables and graphs, calculating averages, and solving real-world problems involving money or unit conversions. Whether you’re brushing up on the basics or tackling more advanced questions, these revision pages are designed to help you build speed, accuracy, and confidence.
Revise for free with our revision topic pages or register for free access to our online numerical reasoning practice tests.

Our Help Articles
Our numerical reasoning test and other education articles are updated regularly to inform you of the latest news and updates across the education sector.